Level Order Tree Traversal

Level order traversal of a tree is breadth first traversal for the tree.

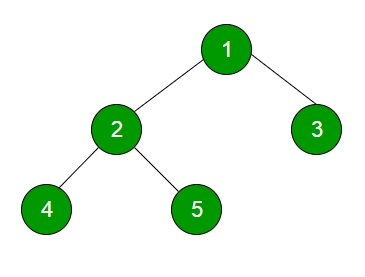
Level order traversal of the above tree is 1 2 3 4 5

METHOD 1 (Use function to print a given level)

Algorithm: There are basically two functions in this method. One is to print all nodes at a given level (printGivenLevel), and other is to print level order traversal of the tree (printLevelorder). printLevelorder makes use of printGivenLevel to print nodes at all levels one by one starting from root.

/*Function to print level order traversal of tree*/
printLevelorder(tree)
for d = 1 to height(tree)
   printGivenLevel(tree, d);

/*Function to print all nodes at a given level*/
printGivenLevel(tree, level)
if tree is NULL then return;
if level is 1, then
    print(tree->data);
else if level greater than 1, then
    printGivenLevel(tree->left, level-1);
    printGivenLevel(tree->right, level-1);

# Recursive Python program for level order traversal of Binary Tree 

# A node structure 
class Node: 

	# A utility function to create a new node 
	def __init__(self, key): 
		self.data = key 
		self.left = None
		self.right = None


# Function to print level order traversal of tree 
def printLevelOrder(root): 
	h = height(root) 
	for i in range(1, h+1): 
		printGivenLevel(root, i) 


# Print nodes at a given level 
def printGivenLevel(root , level): 
	if root is None: 
		return
	if level == 1: 
		print "%d" %(root.data), 
	elif level > 1 : 
		printGivenLevel(root.left , level-1) 
		printGivenLevel(root.right , level-1) 


""" Compute the height of a tree--the number of nodes 
	along the longest path from the root node down to 
	the farthest leaf node 
"""
def height(node): 
	if node is None: 
		return 0
	else : 
		# Compute the height of each subtree 
		lheight = height(node.left) 
		rheight = height(node.right) 

		#Use the larger one 
		if lheight > rheight : 
			return lheight+1
		else: 
			return rheight+1

# Driver program to test above function 
root = Node(1) 
root.left = Node(2) 
root.right = Node(3) 
root.left.left = Node(4) 
root.left.right = Node(5) 

print "Level order traversal of binary tree is -"
printLevelOrder(root) 

#This code is contributed by Nikhil Kumar Singh(nickzuck_007) 

Output:

Level order traversal of binary tree is - 
1 2 3 4 5 

Time Complexity: O(n^2) in worst case. For a skewed tree, printGivenLevel() takes O(n) time where n is the number of nodes in the skewed tree. So time complexity of printLevelOrder() is O(n) + O(n-1) + O(n-2) + .. + O(1) which is O(n^2).

METHOD 2 (Use Queue)

Algorithm: For each node, first the node is visited and then it’s child nodes are put in a FIFO queue.

printLevelorder(tree)
1) Create an empty queue q
2) temp_node = root /*start from root*/
3) Loop while temp_node is not NULL
    a) print temp_node->data.
    b) Enqueue temp_node’s children (first left then right children) to q
    c) Dequeue a node from q and assign it’s value to temp_node

Implementation: Here is a simple implementation of the above algorithm. Queue is implemented using an array with maximum size of 500. We can implement queue as linked list also.

# Python program to print level order traversal using Queue 

# A node structure 
class Node: 
	# A utility function to create a new node 
	def __init__(self ,key): 
		self.data = key 
		self.left = None
		self.right = None

# Iterative Method to print the height of binary tree 
def printLevelOrder(root): 
	# Base Case 
	if root is None: 
		return
	
	# Create an empty queue for level order traversal 
	queue = [] 

	# Enqueue Root and initialize height 
	queue.append(root) 

	while(len(queue) > 0): 
		# Print front of queue and remove it from queue 
		print queue[0].data, 
		node = queue.pop(0) 

		#Enqueue left child 
		if node.left is not None: 
			queue.append(node.left) 

		# Enqueue right child 
		if node.right is not None: 
			queue.append(node.right) 

#Driver Program to test above function 
root = Node(1) 
root.left = Node(2) 
root.right = Node(3) 
root.left.left = Node(4) 
root.left.right = Node(5) 

print "Level Order Traversal of binary tree is -"
printLevelOrder(root) 
#This code is contributed by Nikhil Kumar Singh(nickzuck_007) 

Output:

Level order traversal of binary tree is - 
1 2 3 4 5 

Time Complexity: O(n) where n is number of nodes in the binary tree